Johann Kepler - a Legislator of the Sky.
 The laws of the planets movements
put by Johann Kepler destroyed the thousand year old dogmas about the circles and spheres
and opened the way to scientific understanding of celestial occurrences. The history of
these laws is in Kepler's books. He described all his works there.
The laws of the planets movements
put by Johann Kepler destroyed the thousand year old dogmas about the circles and spheres
and opened the way to scientific understanding of celestial occurrences. The history of
these laws is in Kepler's books. He described all his works there.
Johann Kepler (1571 - 1630) is a german astronomer, one of
the creators of modern astronomy and physics. In 1600 Kepler came to Prague and became an
assistant of the famous Danish astronomer Ticho Brague. Kepler
saw the shortcomings of all exiting systems. Partly that is why he came to Brague in order
to make a new theory on his material. Principles of a new theory he has known already.
After Ticho Brague's death Kepler received a title of the first mathematician of emperor.
The best object for observation was Mars - its orbit unlike
the Earth's one has a noticeable eccentricity, the rotation period in comparison with
Jupiter and Saturn is not too long and observation conditions are better than for Mercury
and Venus.
Kepler proclaimed the planets to be "the globes" as
the Earth (and the first telescopic observations would be only 10 years later). At that
time the only celestial body having probably the terrestrial nature may seemed the Moon,
but the scientist considering the planets system to be a single whole, spread it on the
other planets. With such thoughts Kepler began to develop a theory of Mars movement. He
discovered that the Sun orbit passed through the sun's centre and he constructed Mars's
orbit. But then Kepler came to the conclusion that his founded scheme - equant - is not
true. That is why Kepler decided to study not Mars but the Earth: it was necessary to make
more exact the character of the Earth movement and its orbit, it was necessary to find out
a law of changing the Earth speed. Using the hypothesis of the terrestrial gravity to the
Sun Kepler supposed that the speed must be in increase ratio to the distance from the
Earth to the Sun. This suggestion was true for perihelion (the nearest point to
the Sun) and aphelion (the farthest point). Then Kepler began to check up the
hypothesis for the different parts of the orbit.
Soon it became obviously that the planet passed the equal
distances of the orbit's sectors in equal periods of time. Probably in such a way the
ratio between the planet's speed and its characteristics was discovered at the begining of
1602. The modern formulation of his ratio is called "the second law of Kepler"
(though it had been discovered before the first). Here it is: "Radius-vector of the
planet in the equal intervals of time circumscribes the equal areas".
Kepler calculated Mars's position. Three years Kepler was
searching for Mars orbit form. He used the cut-and-try methods. After a long time of
calculating he came to the conclusion that Mars's orbit is an ellipse. This is the first
law of Kepler. Now it is formulated as follows: "Every planet turns around an
ellipse, in one focus of which is the Sun". Ellipse is a proportionally pressed
circumference.
Kepler tried to find out the mathematic laws of the Solar
system geometry. He seemed up his ideas about numerical and geometrical ratio in his book
"The Harmony of the World". There is the third law of Kepler: "The squares
of the stars periods of planets rotations are as the cube of the big half axle of their
orbits".
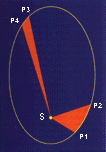
The law of squares illustration. While its rotation around
the Sun (S) the planet (p) does not have the stabble velocity. Near the perihelion point
the velocity is maximum, near the aphelion - it is minimum. Owing to this and to the fact
that the Sun is in one of the focus of an elliptical orbit, the figure, formed by the
radiu-vector SP1, SP2 and another orbit P1P2 and the figure, formed by the radius-vector
SP3, SP4 and the arch P3P4 will have the same squares. |
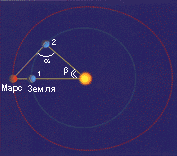
The scheme, used by Kepler for the Earth orbit studing. First
the Sun, the Earth and Mars are in the same straight line. After a period of Mars turning
around the Sun the Earth will take the position 2 and Mars will return in the first
position 1. From Ticho Brague's observations (of the Danish astronomer of the XVI century)
Kepler has found all angless a and b and determined the distance between the Sun and
the Earth in the position 2 in the units ofthe distance between Mars and the Sun. |
All the three Kepler's laws can be applied to the planets
satellites - the natural and artificial. Just Kepler's laws became the basis and
experimental confirmation of new celestial body.
 The laws of the planets movements
put by Johann Kepler destroyed the thousand year old dogmas about the circles and spheres
and opened the way to scientific understanding of celestial occurrences. The history of
these laws is in Kepler's books. He described all his works there.
The laws of the planets movements
put by Johann Kepler destroyed the thousand year old dogmas about the circles and spheres
and opened the way to scientific understanding of celestial occurrences. The history of
these laws is in Kepler's books. He described all his works there.
